Imagine a frying pan coating that a knife can’t scratch. Or truly indestructible steel. Those were just two of the first real-world applications for quasicrystals – a relatively new form of matter discovered only 40 years ago. While the cooking example didn’t pan out (salt, it turns out, was its kryptonite), and quasicrystal-infused steel is currently limited to surgical tools (it can only be produced in small quantities), the possibilities, says Dr. Nicolae Strungaru, are endless.
“We don’t even know the real potential yet – there could be billions of applications for quasicrystals – and we probably won’t for another 100 years.”
The mathematics professor and recipient of MacEwan University’s 2022 Distinguished Research Award is okay with that. He has already spent nearly two decades studying the math behind these almost-crystals that form under extreme conditions that can only be found in space or created in a lab.
Although the complex research that blends mathematics and materials science requires a lot of explanation (more on that later), it’s the simplicity of the mathematical problems quasicrystals present that Dr. Strungaru appreciates.
“In my opinion, there are two types of interesting problems in mathematics. One is a very hard problem with a very short and neat solution. The other is a very short and neat problem, but with a solution that is very hard to find.”
His current work falls into the second category. “The basic mathematical questions we are asking in the field of quasicrystals are pretty elemental,” he says. “But they are very hard problems to solve.”
For a century, explains Dr. Strungaru, scientists understood crystals to be simple configurations of atoms and molecules that are ordered, periodic (repeat in all directions) and have what is called a clear diffraction pattern (if you run radiation through a crystal, it will diffract into a pattern that looks like bright stars on a clear or cloudy night sky). Those clear diffractions could only be produced by crystals with two-, three-, four- or six-fold symmetry. Until 1982, that is, when materials scientist Dan Shechtman discovered a clear diffraction pattern with 10-fold symmetry.
“Quasicrystals have very odd and unique properties – clear diffraction patterns and typically forbidden symmetry,” says Strungaru. “It was very puzzling and contradicted a century of experiments.”
Forty years later, scientists know much more about quasicrystals, but there is still much to learn.
The main goal of Dr. Strungaru’s current research is figuring out how to create mathematical models, called Meyer sets, that are almost periodic – structures that can produce a diffraction pattern which is very clear but repeat at intervals that are imprecise and irregular.
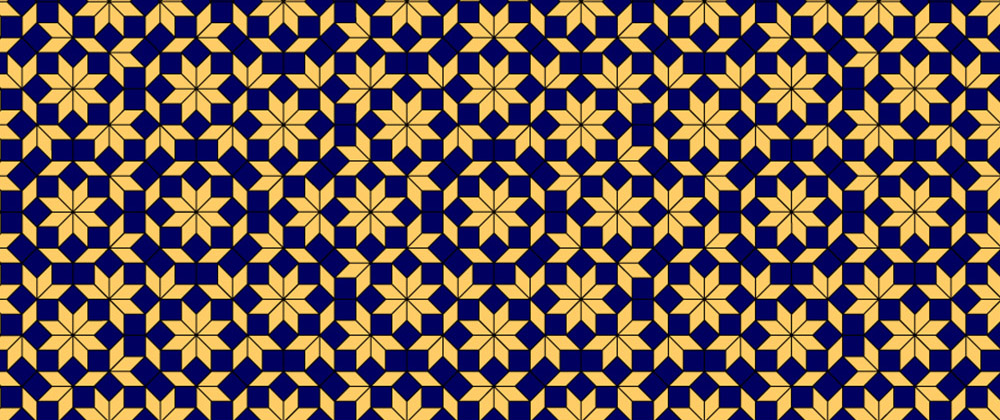
An example of one of Dr. Strungaru’s favourite Meyer sets, called Ammann-Beenker. Source: https://tilings.math.uni-bielefeld.de/substitution/ammann-beenker/
“In some cases, you might have to go for over a kilometre in a single direction to find an overlap in the pattern,” he explains. “This is not exactly periodicity, but almost – so almost periodicity.”
In partnership with European colleagues, Dr. Strungaru has demonstrated a significant connection between almost periodicity and the clear diffraction pattern that quasicrystals create. His solo and co-authored reviews on the subject fill 170 pages in what will be a five-volume work on aperiodic order published by the Cambridge University Press. That series is expected to be the definitive source on the subject for many years to come.
“For me, doing research is pleasure, not work,” says Dr. Strungaru. “And being recognized for my research is a big honour.
It’s also a great motivator.
“It’s significant to receive an award for work I have done in the past, but most important, it’s encouragement to continue this work into the future.”
